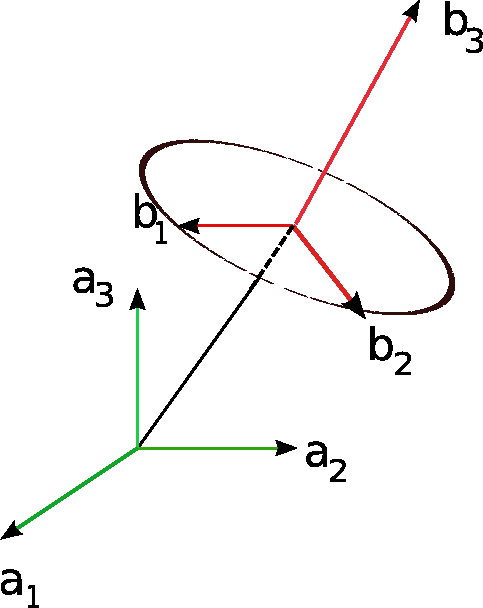
Figure 4↓ shows frame B (the red frame) rotating with respect to frame A (the green frame) about unit vector b₂. One can write the angular velocity as

Notice that b₃ in this case is not equal to a₃. However one can always write b₃ in the A frame using the general form b₃ = α₁ a₁ + α₂ a₂ + α₃ a₃.

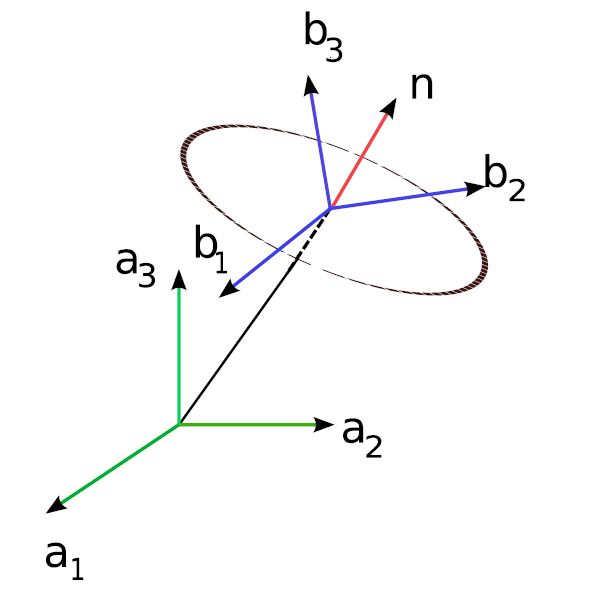
Figure 5↓ shows even a more general case, where the B frame (the blue one) rotates with respect to the A frame about unit vector (n). In this general case there is no such vector as θ, where θ̇ = ω_B ⁄ A. However still one can define the angular velocity of frame B with respect to frame A as ω_B ⁄ A = θ̇n where n can be decomposed in either frame A or B.

Further explanations are provided on how to apply angular velocity to points on a single body or in different reference frames.