STATIC DETERMINACY EXAMPLES

Determine whether the following fixed determinate:

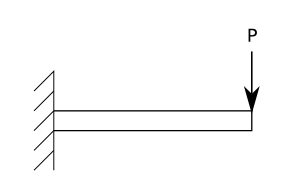
By plotting a free body diagram of the problem above, the number of unknowns can be determined to be less than or equal to the number of equilibrium equations. If this is to be the case, this problem is statically determinate.

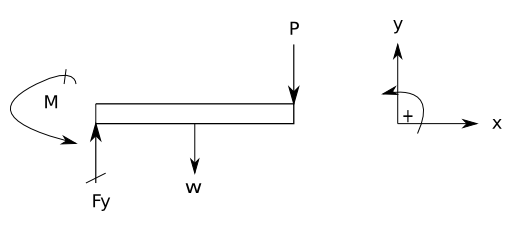
As seen from the picture above, there are two unknown reaction forces caused by both force P and the weight of the beam. Assuming that force P and the weight of the beam are known, one is left with only two unknowns. Since there are 3 equations of equilibrium it is easily understood that this is a statically determinate problem. In fact, one should only have to use the moment equation, ΣM_p = 0, along with the net forces in the y direction, ΣF_y = 0 in order to solve for these unknowns. Notice that there is a horizontal load equal to zero.

Determine whether the following fixed-fixed beam is statically determinate:

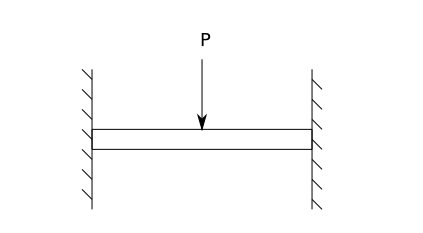
Once again, plot a free body diagram of the problem above and find out if the equations of equilibrium will be enough to solve for its reactions.

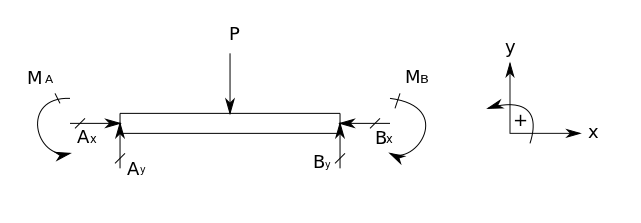
Notice that the equations of equilibrium are not enough for this case, considering there are three equations of equilibrium and six unknowns. One might be wondering why there are six unknowns and not seven. The reason for this is simple. One should assume that force P is a given force. By bringing the constitutive equations one should be able to solve for all of the reaction forces acting on the beam.