Welcome to AE Resources

Planar rotation

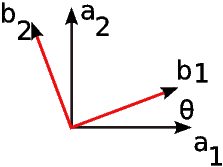
Let frame B (with unit vectors b₁, b₂ and b₃) rotates with respect to A frame (with unit vectors a₁, a₂ and a₃). Figure 0.3 shows a special case when B rotates about b₃ = a₃ with angle θ with respect to A.

Figure 0.3: Planar angular velocity– a₁ -a₂ plane

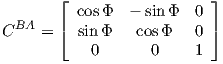⌊ ⌋ cosΦ - sin Φ 0 CBA = ⌈ sinΦ cosΦ 0 ⌉ 0 0 1

(0.2)

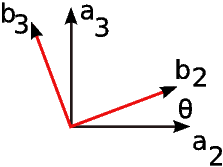
Similarly in the case shown in Fig. 0.4, direction cosine matrix is
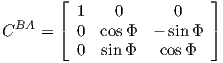$⌊ ⌋ 1 0 0 CBA = ⌈ 0 cosΦ - sin Φ ⌉ 0 sinΦ cosΦ$ (0.3)

Figure 0.4: Planar angular velocity– a₂ -a₃ plane

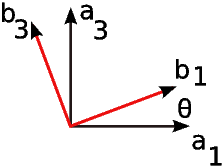
and in the case shown in Fig. 0.5, direction cosine matrix is
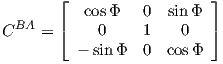$⌊ ⌋ cosΦ 0 sin Φ CBA = ⌈ 0 1 0 ⌉ - sinΦ 0 cosΦ$ (0.4)

Figure 0.5: Planar angular velocity– a₃ -a₁ plane

Any of these cases are called simple rotation.

← Previous Page