|
||||||||
 |
 |
 |
 |
|||||
 |
||||||||
 |
||||||||
 |
 |
|||||||
 |
||||||||
 |
||||||||
 |
||||||||
 |
||||||||
 |
||||||||
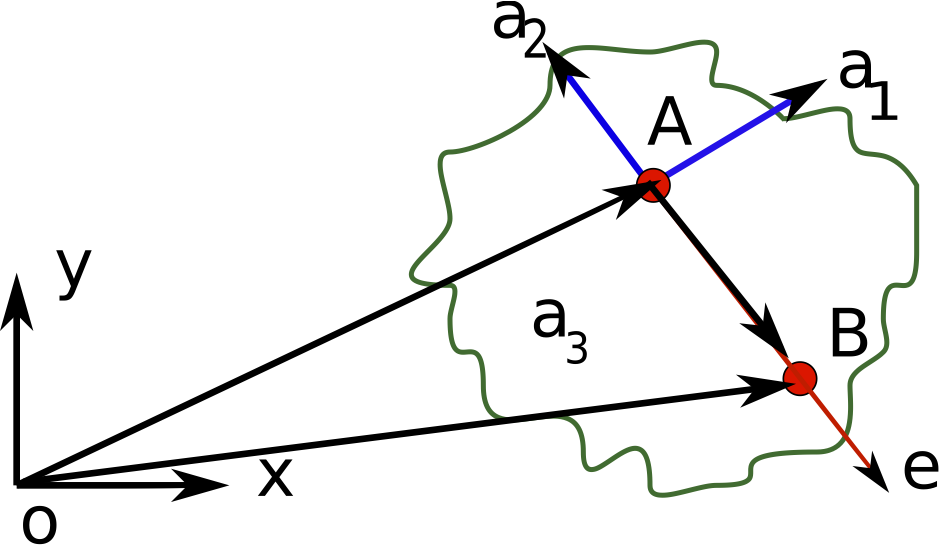
PLANAR VELOCITY OF TWO POINTS ON THE SAME RIGID BODY
Figure 3↓ shows the same problem but simplified to motion in the x − y plane. ωa ⁄ I simplifies to θ̇k where k is the unit vector in a3 or z direction. PAB is a vector in x − y or a1 − a2 plane so that
where the α and β terms represent constants in each coordinate system. Taking the derivatives, and substituting the values from eq. 14↑ the yields
(15)
VB
=
VA + ωa ⁄ I × PAB
VA + θ̇k × (β1 i + β2 j)
=
VA + θ̇β1 j − θ̇β2 i
VA + θ̇a3 × (α1 a1 + α2 a2)
=
VA + θ̇α1 a2 − θ̇α2 a1