Welcome to AE Resources

Converted document Converted document

CYLINDRICAL COORDINATE SYSTEM EXAMPLE

Example 1: Derivation

Derive, without inserting any values for r, θ, and z, the velocity v⃗_P and acceleration a⃗_P equations in cylindrical coordinates of point P from the position vector r⃗_OP.

The position vector r⃗_OP = rê_r + Zk̂ can be written in terms of Cartesian coordinates: x = rcos(θ) and y = rsin(θ) to be

ê_r = cos(θ)î + sin(θ)ĵ

ê_θ = − sin(θ)î + cos(θ)ĵ

Differentiating r⃗_OP gives

v⃗_P = (dr⃗_OP)/(dt) = r⃗̇ = ṙê_r + rê̇_r + Żk̂

Since e⃗_r is relative to P and k̂ is fixed to P:

e⃗̇_r = − θ̇sin(θ)î + θ̇cos(θ)ĵ = θ̇ê_θ

Replacing these unit definitions into the velocity equation gives the velocity:

(1) v⃗_P = ṙê_r + θ̇rê_θ + Żk̂

To find the acceleration, differentiate again:

a⃗_P = v⃗̇_P = r̈ê_r + ṙê̇_r + θ̈rê_θ + θ̇ṙê_θ + θ̇rê̇_θ + Z̈k̂

Replacing the unit vector definitions and noting that ê̇_θ = − θ̇cos(θ)î − θ̇sin(θ)ĵ = − θ̇ê_r, the acceleration can be written as

(2) a⃗_P = (r̈ − rθ̇²)ê_r + (rθ̈ + 2ṙθ̇)ê_θ + Z̈k̂

Example 1: Application

A helicopter, ’B’, flies a helical pattern as shown in the figure. The vertical drop for each revolution (2π) as θ changes on the helix can be written as (pθ)/(2π).

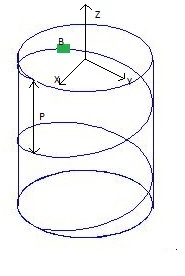
Determine the position, velocity and acceleration of point B in cylindrical coordinates. For the values R = 0.3m, p = 0.2m, and θ̇ = 0.6t(rad)/(s), find the values for the velocity and acceleration vectors of B when t = 10s.

Consider the location of the helicopter in time. Here r⃗ = Rê_r *.5cmz⃗ = − (Pθ)/(2π)k̂ where θ = θ(t).

The position vector at any time can be written as:

(3) r⃗_OB = Re⃗_r + ⎛⎝ − (Pθ)/(2π)⎞⎠k̂

Similarly, from eqn. 1↑, the velocity of the helicopter is

v⃗_B = Ṙê_r + θ̇Rê_θ + ⎛⎝ − (Pθ̇)/(2π)⎞⎠k̂