Consider the following example problem.

A drop of water, D, is moving towards the center of a disk, B, of radius, r = 0.5ft that is rotating with a constant angular velocity with respect to a fixed frame of reference frame G. The disk is mounted on a bar of 1 ft. that is also rotating at a constant angular velocity. The drop of water is moving at a velocity of 0.02 ft/sec and has an acceleration of 0.01 ft/sec/sec.

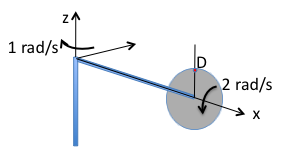
The angular velocity of the disk in the fixed frame of reference is a combination of the two rotations:

The velocity of the drop of water D with respect to G can be written as V_(D)/(G) = V_(D)/(B) + V_(O’)/(G) + (ω_(D)/(G) × r_O’D) where O’ is the center of the disk.

The drop of water is moving downward on the disk, so V_(D)/(B) =  − 0.02 k. The velocity of the disk with respect to the reference frame is V_(O’)/(G) is a rotation about the fixed point on the bar, so the velocity is the angular velocity times the radius or ( − 1)(1) j (the  − 1 is the angular velocity). The distance of the drop is currently the radius of the disk, and the drop is in the positive z direction, so r_O’D = 0.5 k. Substituting these values